SLOPE—A MEASURE OF STEEPNESS 7.1.3 through 7.1.5

Algebra Connections Parent Guide

50

2 4–2–4

2

4

–2

–4

x

y

2 4–2–4

2

4

–2

–4

x

y

2 4–2–4

2

4

–2

–4

x

y

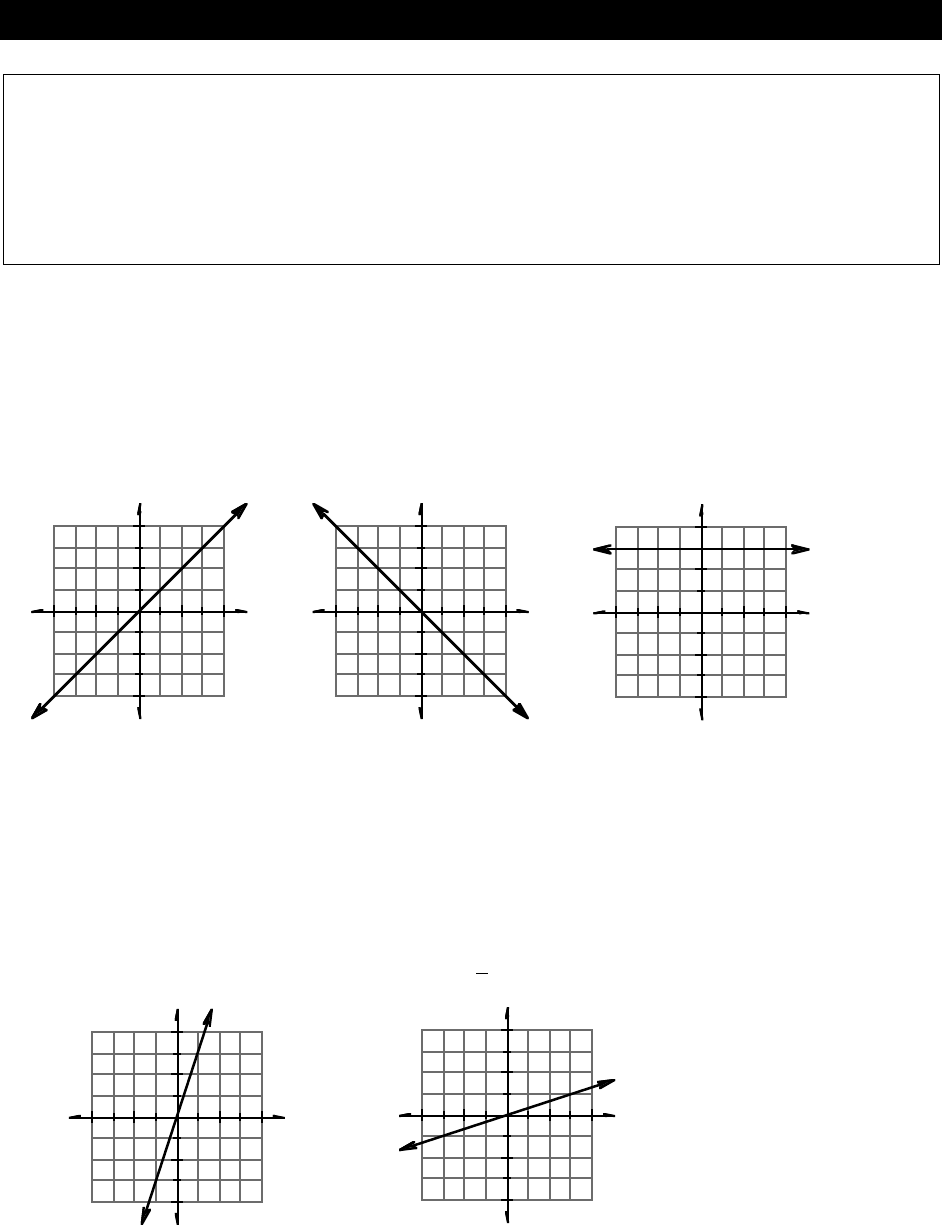
SLOPE—A MEASURE OF STEEPNESS 7.1.3 through 7.1.5

Students have used the equation

y = mx + b

throughout this course to graph lines and

describe patterns. When the equation is written in y-form, the m is the coefficient of x

and is the slope of the line. It indicates the direction of the line and its steepness. The

constant, b, is the y-intercept, written (0, b), and indicates where the line crosses the

y-axis. See the Math Notes boxes on pages 283, 291, and 298.

Example 1

If m is positive, the line goes upward from left to right. If m is negative, the line goes downward

from left to right. If m = 0 then the line is horizontal.

y = x

y = !x

y = 0x + 3

or

y = 3

Example 2

When

m = 1

, as in

y = x

, the line goes upward by one unit each time it goes over one unit to the

right. Steeper lines have a larger m value, that is,

m > 1

. Flatter lines have an m value between

0 and 1, usually in the form of a fraction.

y = 3x

y =

1

3

x

2 4–2–4

2

4

–2

–4

x

y

2 4–2–4

2

4

–2

–4

x

y

Chapter 7: Linear Relationships

51

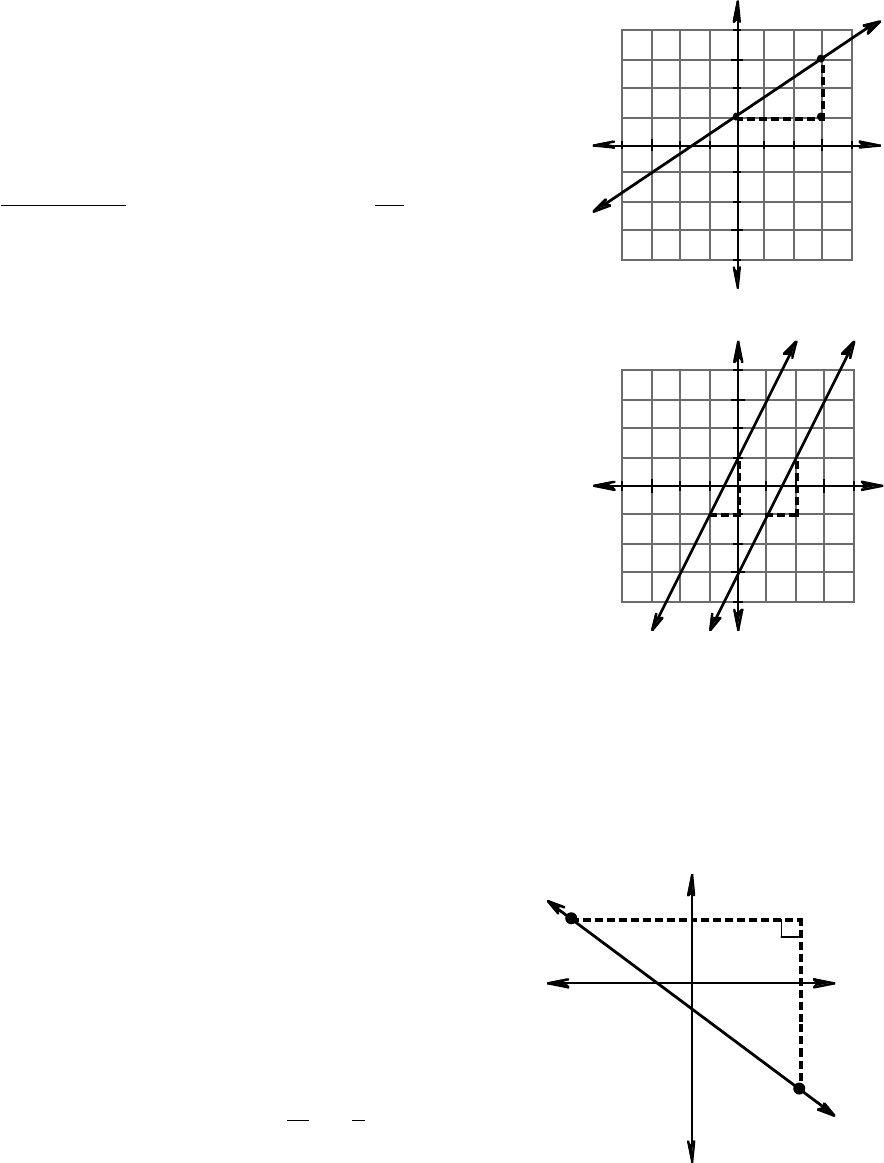
Example 3

Slope is written as a ratio. If the line is drawn on a set of axes,

a slope triangle can be drawn between any two convenient

points (that is, where grid lines cross and coordinates are

integers), as shown in the graph at right. Count the vertical

distance and the horizontal distance on the dashed sides of the

slope triangle. Write the distances in a ratio:

m =

vertical! !

horizontal!!

. Another form used is:

!y

!x

. The symbol

!

means change. The order in the fraction is important: the

numerator (top of the fraction) must be the vertical distance

and the denominator (bottom of the fraction) must be the

horizontal distance.

Parallel lines have the same steepness and direction, so they

have the same slope, as shown in the graph at right.

If

!y = 0

, then the line is horizontal and has a slope of zero,

that is,

m = 0

. If

!x = 0

, then the line is vertical and its slope is

undefined, so we say that it has no slope.

Example 4

When the vertical and horizontal distances are not easy to determine, you can find the slope by

drawing a generic slope triangle and using it to find the lengths of the vertical (

!y

) and

horizontal (

!x

) segments. The figure at right shows how to find the slope of the line that passes

through the points (-21, 9) and (19, -15). First graph the

points on unscaled axes by approximating where they are

located, then draw a slope triangle. Next find the distance

along the vertical side by noting that it is 9 units from point

B to the x-axis then 15 units from the x-axis to point C, so

!y

is 24. Then find the distance from point A to the y-axis

(21) and the distance from the y-axis to point B (19).

!x

is

40. This slope is negative because the line goes downward

from left to right, so the slope is

!

24

40

= !

3

5

.

3–3

3

–3

y

x

y = 2x ! 3,! y = 2x + 1

!y = 2

!x = 3

3–3

3

–3

y

x

A

B

(-21,9)

(19,-15)(19,-15)

C

!x

=40

!y

=24

Algebra Connections Parent Guide

52

Problems

Is the slope of these lines negative, positive or zero?

1.

y

x

y

x

2.

y

x

y

x

3.

y

x

y

x

Identify the slope in these equations. State whether the graph of the line is steeper or flatter than

y = x

or

y = ! x

, whether it goes up or down from left to right, or if it is horizontal or vertical.

4.

y = 3x + 2

5.

y = !

1

2

x + 4

6.

y =

1

3

x ! 4

7.

4 x ! 3 = y

8.

y = !2 +

1

2

x

9.

3 + 2y = 8x

10.

y = 2

11.

x = 5

12.

6x + 3y = 8

Without graphing, find the slope of each line based on the given information.

13.

!y = 27! !x = "8

14.

!x = 15! !y = 3

15.

!y = 7!!x = 0

16.

Horizontal

! = 6

Vertical

! = 0

17.

Between (5, 28) and

(64, 12)

18.

Between (-3, 2) and

(5, -7)

Answers

1.

zero

2.

negative

3.

positive

4.

Slope = 3, steeper, up

5.

Slope = -

1

2

, flatter, down

6.

Slope =

1

3

, flatter, up

7.

Slope = 4, steeper, up

8.

Slope =

1

2

, flatter, up

9.

Slope = 4, steeper, up

10.

horizontal

11.

vertical

12.

Slope = -2, steeper, down

13.

!

27

8

14.

3

15

=

1

5

15.

undefined

16.

0

17.

!

16

59

18.

!

9

8

Chapter 7: Linear Relationships

53

WRITING AN EQUATION GIVEN THE SLOPE 7.3.1 and 7.3.2

AND A POINT ON THE LINE

In earlier work students used substitution in equations like

y = 2x + 3

to find x and y

pairs that make the equation true. Students recorded those pairs in a table, then used

them as coordinates to graph a line. Every point (x, y) on the line makes the equation

true.

Later in the course, students used the patterns they saw in the tables and graphs to

recognize and write equations in the form of

y = mx + b

. The “b” represents the

y-intercept of the line, the “m” represents the slope, while x and y represent the

coordinates of any point on the line. Each line has a unique value for m and a unique

value for b, but there are infinite (x, y) values for each linear equation. The slope of the

line is the same between any two points on that line. We can use this information to

write equations without creating tables or graphs. See the Math Notes box on page 308.

Example 1

What is the equation of the line with a slope of 2 that passes through the point (10, 17)?

Write the general equation of a line.

Substitute the values we know: m, x, and y.

Solve for b.

Write the complete equation using the values

of m (2) and b (-3).

y = mx + b

17 = 2(10 ) + b

17 = 20 + b

!3 = b

y = 2x ! 3

Algebra Connections Parent Guide

54

Example 2

This algebraic method can help us write equations of parallel and perpendicular lines. Parallel

lines never intersect or meet. They have the same slope, m, but different y-intercepts, b.

What is the equation of the line parallel to

y = 3x ! 4

that goes through the point (2, 8)?

Write the general equation of a line.

y = mx + b

Substitute the values we know: m, x, and y.

Since the lines are parallel, the slopes are equal.

8 = 3(2) + b

Solve for b.

8 = 6 + b

2 = b

Write the complete equation.

y = 3x + 2

Example 3

Perpendicular lines meet at a right angle, that is,

90°

. They have opposite, reciprocal slopes

and may have different y-intercepts. If one line is steep and goes upward, then the line

perpendicular to it must be shallow and go downward.

Reciprocals are pairs of numbers that when multiplied equal one. For example, 3 and

1

3

are

reciprocals as are

5

2

and

2

5

. Opposites have different signs: positive or negative. Examples of

opposite reciprocal slopes are

!

1

5

and 5;

!

2

3

and

3

2

; and

!2

and

1

2

.

Write the equation of the line that is perpendicular to

y = 2x + 4

and goes through the point

(6, 3).

Write the general equation of a line.

y = mx + b

Substitute the values we know: m, x, and y.

Since the line is perpendicular, the new slope is

!

1

2

.

3 = !

1

2

(6) + b

Solve for b.

3 = !3 + b

6 = b

Write the complete equation.

y = !

1

2

x + 6

Chapter 7: Linear Relationships

55

Problems

Write the equation of the line with the given slope that passes through the given point.

1.

slope = 5, (3, 13)

2.

slope =

!

5

3

, (3, -1)

3.

slope = -4 , (-2, 9)

4.

slope =

3

2

, (6, 8)

5.

slope = 3, (-7, -23)

6.

slope = 2, (

5

2

, -2)

Write the equation of the line parallel to the given line that goes through the given point.

7.

y =

3

5

x + 2

(0, 0)

8.

y = 4 x ! 1

(-2, -6)

9.

y = !2 x + 5

(-4, -2)

10.

y = 4 x + 5

(-6, -28)

11.

y =

1

3

x ! 1

(6, 9)

12.

y = 3x + 8

(0,

1

2

)

Write the equation of the line perpendicular to the given line that goes through the given point.

13.

y =

3

5

x + 2

(0, 0)

14.

y = 4 x ! 1

(-2, 6)

15.

y = !2 x + 5

(-4, -2)

16.

y =

1

3

x ! 1

(6, -2)

17.

y = 4 x + 5

(20, -28)

18.

y = 3x +

1

2

(0,

1

2

)

Answers

1.

y = 5 x ! 2

2.

y = !

5

3

x + 4

3.

y = !4 x + 1

4.

y =

3

2

x ! 1

5.

y = 3x ! 2

6.

y = 2x ! 7

7.

y =

3

5

x

8.

y = 4 x + 2

9.

y = !2x ! 10

10.

y = 4 x ! 4

11.

y =

1

3

x + 7

12.

y = 3x +

1

2

13.

y = !

5

3

x

14.

y = !

1

4

x + 5

1

2

15.

y =

1

2

x

16.

y = !3x + 16

17.

y = !

1

4

x ! 23

18.

y = !

1

3

x +

1

2

Algebra Connections Parent Guide

56

WRITING THE EQUATION OF A LINE GIVEN TWO POINTS 7.3.3

Students now have all the tools they need to find the equation of a line passing through

two given points. Recall that the equation of a line requires a slope and a y-intercept in

y = mx + b

. Students can write the equation of a line from two points by creating a slope

triangle and calculating

!y

!x

as explained in sections 7.1.3 through 7.1.5. See the Math

Notes box on page 314.

Example 1

Write the equation of the line that passes through the points (1, 9) and (-2, -3).

Draw a generic slope triangle.

Calculate the slope using the given two points.

Write the general equation of a line.

y = mx + b

Substitute m and one of the points for x and y,

in this case (1, 9).

9 = 4(1) + b

Solve for b.

5 = b

Write the complete equation.

y = 4 x + 5

Example 2

Write the equation of the line that passes through the points (8, 3) and (4, 6).

Draw a generic slope triangle.

Calculate the slope using the given two points.

Write the general equation of a line.

y = mx + b

Substitute m and one of the points for x and y,

in this case (8, 3).

3 = !

3

4

(8) + b

Solve for b.

3 = !6 + b

9 = b

Write the complete equation.

y = !

3

4

x + 9

(1, 9)

(-2, -3)

12

3

!y

!x

=

12

3

,

m = 4

(4, 6)

(8, 3)

4

3

!y

!x

=

3

4

,

m = "

3

4

Chapter 7: Linear Relationships

57

Problems

Write the equation of the line containing each pair of points.

1.

(1, 1) and (0, 4)

2.

(5, 4) and (1, 1)

3.

(1, 3) and (-5, -15)

4.

(-2, 3) and (3, 5)

5.

(2, -1) and (3, -3)

6.

(4, 5) and (-2, -4)

7.

(1, -4) and (-2, 5)

8.

(-3, -2) and (5, -2)

9.

(-4, 1) and (5, -2)

Answers

1.

y = !3x + 4

2.

y =

3

4

x +

1

4

3.

y = 3x

4.

y =

2

5

x + 3

4

5

5.

y = !2x + 3

6.

y =

3

2

x ! 1

7.

y = !3x ! 1

8.

y = !2

9.

y = !

1

3

x !

1

3